It was six men of Indostan, to learning much inclined,
who went to see the elephant (Though all of them were blind),
that each by observation, might satisfy his mind.
The first approached the elephant, and, happening to fall,
against his broad and sturdy side, at once began to bawl:
'God bless me! but the elephant, is nothing but a wall!'
The second feeling of the tusk, cried: 'Ho! what have we here,
so very round and smooth and sharp? To me tis mighty clear,
this wonder of an elephant, is very like a spear!'
The third approached the animal, and, happening to take,
the squirming trunk within his hands, 'I see,' quoth he,
the elephant is very like a snake!'
The fourth reached out his eager hand, and felt about the knee:
'What most this wondrous beast is like, is mighty plain,' quoth he;
'Tis clear enough the elephant is very like a tree.'
The fifth, who chanced to touch the ear, Said; 'E'en the blindest man
can tell what this resembles most; Deny the fact who can,
This marvel of an elephant, is very like a fan!'
The sixth no sooner had begun, about the beast to grope,
than, seizing on the swinging tail, that fell within his scope,
'I see,' quothe he, 'the elephant is very like a rope!'
And so these men of Indostan, disputed loud and long,
each in his own opinion, exceeding stiff and strong,
Though each was partly in the right, and all were in the wrong!
So, oft in theologic wars, the disputants, I ween,
tread on in utter ignorance, of what each other mean,
and prate about the elephant, not one of them has seen!
John Godfrey Saxe

- Six blind men describe an elephant.jpg (49.22 KiB) Viewed 41361 times
Thank you CANAG for trying to make Willreifer see some sense. Unfortunately he has missed the point and still does not appear to understand two fundamental facts concerning the vector of gravitational force and its line of action.
1. Gravitational force in the gravitational field of the earth at all times produces an acceleration of approximately 9.81 m/s/s in the downward direction
(local position on the planet does influence the exact acceleration magnitude, but for practical purposes this variation will have very minor effect upon a pole vaulter).2.
The gravity vector does not possess, by definition, a component in the horizontal direction.
The so called calculations of the potential energy of a vaulter being calculated
on the basis of an average horizontal velocity in the 10m to 5m final interval of the approach run CAN NOT BE the actual VELOCITY or its DIRECTION of any vaulter at the instant of take-off.Also the potential energy (PE) of the vaulter's centre of mass (COM) above the ground must be included in the PE calculation as does the PE gain of the COM from it's height at pole release to its peak height at which point the vaulter's potential energy above the take-off surface will be a maximum.
In terms of the total energy at peak height their will be some energy required to continue to translate and rotate the vaulter that must be factored into the energy calculation.
Total energy, or any energy comparisons, to determine efficiencies between the vaulter must use the ratio of energy per unit body mass (J/kg) and not the absolute value (J).Correlation of Average approach run velocity to successful vault height clearance is an indication of the relationship between the two numbers. A correlation does not mean the relationship is a causative one. Indeed in the homogeneous population of male and female pole vaulters the amount of variation in the numbers (r x r) explained is relatively small and insufficient to be predictive of the outcome of the vault clearance height. Technical and Psychological factors are much greater determinants of the results outcome in comparison to average horizontal final approach velocity for elite vaulters!
No vaulter can have an efficiency of 100%, let alone greater than 100%. To do this a vaulter would be defying the Laws of Conservation of Energy and the Second Law of Thermodynamics! I think vaulting coaches are more concerned with continuous motion, and not perpetual motion, in the pole deflection and recoil phases of real world pole vaulting.
The attempt to show that Vaulter A is superior to Vaulter B using contrived / bogus data and a limited perspective as to the mechanics of pole vaulting is not only time wasting for coaches who want to understand the event but is irritating, especially when ill-informed and irrational claims are being made based on conjecture and mind games by some who are as silly as the men of Indostan!
By the way it just so happens that Bubka and Trandenkov have been studied in the same competition by reputable scientists and in my next post I will put up some of the data they reported in regard to energy exchanges in their vaults on that occasion. In regard to pre-bending the pole by Dossevi (in particular) and the assertions concerning the uniquesness of Lavillenies vaulting technique ,I have some factual matter to put up for readers to consider in judging the veracity of some assertions being made by Willreifer and others.
I leave readers with real data from a real jump by Mike Tully in 1978. Mike Tully ,I believe, could be considered as a pioneer continuous "swinger" throughout the pole deflection and recoil support phase of the vault.
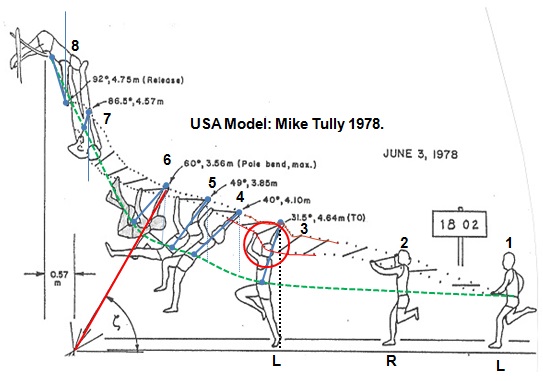
- Mike Tulley take-off pole bending data diagram.jpg (57.41 KiB) Viewed 41361 times
Many coaches in the USA, France, Poland, Former East Germany and the Soviet Union around this time were very aware of the necessity to "swing continuously with vigour throughout the pole support phases" but had not fully understood the mechanism of bending the pole with minimal energy losses and successfully finish the pole plant and take-off. The Petrov - Bubka revolution in the approach run, pole plant and take-off technique was being revealed to the world 1983-84. The key elements were identified in the Petrov seminal paper in Birmingham, England in 1985. The rest is history.
If this thread is anything to go by the lessons of the past history of flexible pole vaulting have still not been understood, let alone practiced.
Is it me... but Willreifer's contributions, as the weeks go by, appear to me to bear more and more resemblance to those of PVdaddy but with less abrasive "attitude"? Some of the questions, purportedly from pm's appeared suspiciously like Dorothy Dixers!